IB Questionbank

User interface language: English | Español

HL Paper 2

An ice-skater is skating such that her position vector when viewed from above at time $t$ seconds can be modelled by

$(\begin{matrix} x \\ y \end{matrix}) = (\begin{matrix} a e^{b t} \cos t \\ a e^{b t} sin t \end{matrix})$

with respect to a rectangular coordinate system from a point $O$ , where the non-zero constants $a$ and $b$ can be determined. All distances are in metres.

At time $t = 0$ , the displacement of the ice-skater is given by $(\begin{matrix} 5 \\ 0 \end{matrix})$ and the velocity of the ice‑skater is given by $(\begin{matrix} - 3.5 \\ 5 \end{matrix})$ .

Find the velocity vector at time $t$ .

[3]

Show that the magnitude of the velocity of the ice-skater at time $t$ is given by

$a e^{b t} \sqrt{(1 + b^{2})}$ .

[4]

Find the value of $a$ and the value of $b$ .

[3]

Find the magnitude of the velocity of the ice-skater when $t = 2$ .

[2]

At a point $P$ , the ice-skater is skating parallel to the $y$ -axis for the first time.

Find $OP$ .

[6]

Markscheme

use of product rule (M1)

$(\begin{matrix} \dot{x} \\ \dot{y} \end{matrix}) = (\begin{matrix} a b e^{b t} \cos t - a e^{b t} sin t \\ a b e^{b t} sin t + a e^{b t} \cos t \end{matrix})$ A1A1

[3 marks]

${|v|}^{2} = {\dot{x}}^{2} + {\dot{y}}^{2} = {[a b e^{b t} \cos t - a e^{b t} sin t]}^{2} + {[a b e^{b t} sin t + a e^{b t} \cos t]}^{2}$ M1

Note: It is more likely that an expression for $|v|$ is seen.
$\sqrt{{\dot{x}}^{2} + {\dot{y}}^{2}}$ is not sufficient to award the M1, their part (a) must be substituted.

$= [a^{2} \sin^{2} t - 2 a^{2} b \sin t \cos t + a^{2} b^{2} \cos^{2} t + a^{2} \cos^{2} t + 2 a^{2} b \sin t \cos t + a^{2} b^{2} \sin^{2} t] e^{2 b t}$ A1

use of $\sin^{2} t + \cos^{2} t = 1$ within a factorized expression that leads to the final answer M1

$= a^{2} (b^{2} + 1) e^{2 b t}$ A1

magnitude of velocity is $a e^{b t} \sqrt{(1 + b^{2})}$ AG

[4 marks]

when $t = 0, a e^{b t} \cos t = 5$

$a = 5$ A1

$a b e^{b t} \cos t - a e^{b t} \sin t = - 3.5$ (M1)

$b = - 0.7$ A1

Note: Use of $a e^{b t} \sqrt{(1 + b^{2})}$ result from part (b) is an alternative approach.

[3 marks]

$5 e^{- 0.7 \times 2} \sqrt{(1 + {(- 0.7)}^{2})}$ (M1)

$1.51 (1.50504 \dots)$ A1

[2 marks]

$\dot{x} = 0$ (M1)

$a e^{b t} (b \cos t - \sin t) = 0$

$\tan t = b$

$t = 2.53 (2.53086 \dots)$ (A1)

correct substitution of their $t$ to find $x$ or $y$ (M1)
$x = - 0.697 (- 0.696591 \dots)$ and $y = 0.488 (0.487614 \dots)$ (A1)

use of Pythagoras / distance formula (M1)
$OP = 0.850 m (0.850297 \dots)$ A1

[6 marks]

Examiners report

[N/A]

A flying drone is programmed to complete a series of movements in a horizontal plane relative to an origin $O$ and a set of $x$ - $y$ -axes.

In each case, the drone moves to a new position represented by the following transformations:

a rotation anticlockwise of $\frac{π}{6}$ radians about $O$
a reflection in the line $y = \frac{x}{\sqrt{3}}$
a rotation clockwise of $\frac{π}{3}$ radians about $O$ .

All the movements are performed in the listed order.

Write down each of the transformations in matrix form, clearly stating which matrix represents each transformation.

[6]

a.i.

Find a single matrix $P$ that defines a transformation that represents the overall change in position.

[3]

a.ii.

Find $P^{2}$ .

[1]

a.iii.

Hence state what the value of $P^{2}$ indicates for the possible movement of the drone.

[2]

a.iv.

Three drones are initially positioned at the points $A$ , $B$ and $C$ . After performing the movements listed above, the drones are positioned at points $A'$ , $B'$ and $C'$ respectively.

Show that the area of triangle $ABC$ is equal to the area of triangle $A' B' C'$ .

[2]

Find a single transformation that is equivalent to the three transformations represented by matrix $P$ .

[4]

Markscheme

Note: For clarity, exact answers are used throughout this markscheme. However it is perfectly acceptable for candidates to write decimal values $(e.g. \frac{\sqrt{3}}{2} = 0.866)$ .

rotation anticlockwise $\frac{π}{6}$ is $(\begin{matrix} 0.866 & - 0.5 \\ 0.5 & 0.866 \end{matrix})$ OR $(\begin{matrix} \frac{\sqrt{3}}{2} & - \frac{1}{2} \\ \frac{1}{2} & \frac{\sqrt{3}}{2} \end{matrix})$ (M1)A1

reflection in $y = \frac{x}{\sqrt{3}}$

$\tan θ = \frac{1}{\sqrt{3}}$ (M1)

$\Rightarrow 2 θ = \frac{π}{3}$ (A1)

matrix is $(\begin{matrix} 0.5 & 0.866 \\ 0.866 & - 0.5 \end{matrix})$ OR $(\begin{matrix} \frac{1}{2} & \frac{\sqrt{3}}{2} \\ \frac{\sqrt{3}}{2} & - \frac{1}{2} \end{matrix})$ A1

rotation clockwise $\frac{π}{3}$ is $(\begin{matrix} 0.5 & 0.866 \\ - 0.866 & 0.5 \end{matrix})$ OR $(\begin{matrix} \frac{1}{2} & \frac{\sqrt{3}}{2} \\ - \frac{\sqrt{3}}{2} & \frac{1}{2} \end{matrix})$ A1

[6 marks]

a.i.

Note: For clarity, exact answers are used throughout this markscheme. However it is perfectly acceptable for candidates to write decimal values $(e.g. \frac{\sqrt{3}}{2} = 0.866)$ .

an attempt to multiply three matrices (M1)

$P = (\begin{matrix} \frac{1}{2} & \frac{\sqrt{3}}{2} \\ - \frac{\sqrt{3}}{2} & \frac{1}{2} \end{matrix}) (\begin{matrix} \frac{1}{2} & \frac{\sqrt{3}}{2} \\ \frac{\sqrt{3}}{2} & - \frac{1}{2} \end{matrix}) (\begin{matrix} \frac{\sqrt{3}}{2} & - \frac{1}{2} \\ \frac{1}{2} & \frac{\sqrt{3}}{2} \end{matrix})$ (A1)

$P = (\begin{matrix} \frac{\sqrt{3}}{2} & - \frac{1}{2} \\ - \frac{1}{2} & - \frac{\sqrt{3}}{2} \end{matrix})$ OR $(\begin{matrix} 0.866 & - 0.5 \\ - 0.5 & - 0.866 \end{matrix})$ A1

[3 marks]

a.ii.

Note: For clarity, exact answers are used throughout this markscheme. However it is perfectly acceptable for candidates to write decimal values $(e.g. \frac{\sqrt{3}}{2} = 0.866)$ .

$(P^{2} = (\begin{matrix} \frac{\sqrt{3}}{2} & - \frac{1}{2} \\ - \frac{1}{2} & - \frac{\sqrt{3}}{2} \end{matrix}) (\begin{matrix} \frac{\sqrt{3}}{2} & - \frac{1}{2} \\ - \frac{1}{2} & - \frac{\sqrt{3}}{2} \end{matrix}) =) (\begin{matrix} 1 & 0 \\ 0 & 1 \end{matrix})$ A1

Note: Do not award A1 if final answer not resolved into the identity matrix $I$ .

[1 mark]

a.iii.

Note: For clarity, exact answers are used throughout this markscheme. However it is perfectly acceptable for candidates to write decimal values $(e.g. \frac{\sqrt{3}}{2} = 0.866)$ .

if the overall movement of the drone is repeated A1

the drone would return to its original position A1

[2 marks]

a.iv.

Note: For clarity, exact answers are used throughout this markscheme. However it is perfectly acceptable for candidates to write decimal values $(e.g. \frac{\sqrt{3}}{2} = 0.866)$ .

METHOD 1

$|det P| = |(- \frac{3}{4}) - (\frac{1}{4})| = 1$ A1

area of triangle $ABC =$ area of triangle $A' B' C'$ $\times |det P|$ R1

area of triangle $ABC =$ area of triangle $A' B' C'$ AG

Note: Award at most A1R0 for responses that omit modulus sign.

METHOD 2

statement of fact that rotation leaves area unchanged R1

statement of fact that reflection leaves area unchanged R1

area of triangle $ABC =$ area of triangle $A' B' C'$ AG

[2 marks]

Note: For clarity, exact answers are used throughout this markscheme. However it is perfectly acceptable for candidates to write decimal values $(e.g. \frac{\sqrt{3}}{2} = 0.866)$ .

attempt to find angles associated with values of elements in matrix $P$ (M1)

$(\begin{matrix} \frac{\sqrt{3}}{2} & - \frac{1}{2} \\ - \frac{1}{2} & - \frac{\sqrt{3}}{2} \end{matrix}) = (\begin{matrix} \cos (- \frac{π}{6}) & \sin (- \frac{π}{6}) \\ \sin (- \frac{π}{6}) & - \cos (- \frac{π}{6}) \end{matrix})$

reflection (in $y = (\tan θ) x$ ) (M1)

where $2 θ = - \frac{π}{6}$ A1

reflection in $y = \tan (- \frac{π}{12}) x (= - 0.268 x)$ A1

[4 marks]

Examiners report

There were many good attempts at this problem. In most cases these good attempts were undermined by a key lack of understanding. Whilst candidates were able to find the correct matrices in part (a)(i), they then invariably went onto multiply the matrices in the wrong order in part (a)(ii). Whilst follow through marks were readily available after this, the incorrect matrix for $P$ then caused issues in part (c). If these candidates had multiplied correctly, it seems that many of them could have gained close to full marks on this question. At the same time there was a lack of precision in the description of the transformation in part (c). As a general point, it would also help candidates if they resolved the trig ratios in the matrices; writing $0.5$ or $\frac{1}{2}$ rather than, for example $\cos (\frac{π}{3})$ . Finally, there were many attempts in part (c) that suggested candidates had a good knowledge and understanding of the concepts of matrices and affine transformations.

a.i.

a.ii.

a.iii.

a.iv.

A ball is attached to the end of a string and spun horizontally. Its position relative to a given point, $O$ , at time $t$ seconds, $t \geq 0$ , is given by the equation

$r = (\begin{matrix} 1.5 \cos (0.1 t^{2}) \\ 1.5 \sin (0.1 t^{2}) \end{matrix})$ where all displacements are in metres.

The string breaks when the magnitude of the ball’s acceleration exceeds $20 {ms}^{- 2}$ .

Show that the ball is moving in a circle with its centre at $O$ and state the radius of the circle.

[4]

Find an expression for the velocity of the ball at time $t$ .

[2]

b.i.

Hence show that the velocity of the ball is always perpendicular to the position vector of the ball.

[2]

b.ii.

Find an expression for the acceleration of the ball at time $t$ .

[3]

c.i.

Find the value of $t$ at the instant the string breaks.

[3]

c.ii.

How many complete revolutions has the ball completed from $t = 0$ to the instant at which the string breaks?

[3]

c.iii.

Markscheme

* This sample question was produced by experienced DP mathematics senior examiners to aid teachers in preparing for external assessment in the new MAA course. There may be minor differences in formatting compared to formal exam papers.

$|r| = \sqrt{1 . 5^{2} \cos^{2} (0.1 t^{2}) + 1 . 5^{2} \sin^{2} (0.1 t^{2})}$ M1

$= 1.5$ as $\sin^{2} θ + \cos^{2} θ = 1$ R1

Note: use of the identity needs to be explicitly stated.

Hence moves in a circle as displacement from a fixed point is constant. R1

Radius $= 1.5 m$ A1

[4 marks]

$v = (\begin{matrix} - 0.3 t \sin (0.1 t^{2}) \\ 0.3 t \cos (0.1 t^{2}) \end{matrix})$ M1A1

Note: M1 is for an attempt to differentiate each term

[2 marks]

b.i.

$v ∙ r = (\begin{matrix} 1.5 \cos (0.1 t^{2}) \\ 1.5 \sin (0.1 t^{2}) \end{matrix}) ∙ (\begin{matrix} - 0.3 t \sin (0.1 t^{2}) \\ 0.3 t \cos (0.1 t^{2}) \end{matrix})$ M1

Note: M1 is for an attempt to find $v ∙ r$

$= 1.5 \cos (0.1 t^{2}) \times (- 0.3 t \sin (0.1 t^{2})) + 1.5 \sin (0.1 t^{2}) \times 0.3 t \sin (0.1 t^{2}) = 0$ A1

Hence velocity and position vector are perpendicular. AG

[2 marks]

b.ii.

$a = (\begin{matrix} - 0.3 \sin (0.1 t^{2}) - 0.06 t^{2} \cos (0.1 t^{2}) \\ 0.3 \cos (0.1 t^{2}) - 0.06 t^{2} \sin (0.1 t^{2}) \end{matrix})$ M1A1A1

[3 marks]

c.i.

${(- 0.3 \sin (0.1 t^{2}) - 0.06 t^{2} \cos (0.1 t^{2}))}^{2} + {(0.3 \cos (0.1 t^{2}) - 0.06 t^{2} \sin (0.1 t^{2}))}^{2} = 400$ (M1)(A1)

Note: M1 is for an attempt to equate the magnitude of the acceleration to $20$ .

$t = 18.3 (18.256 \dots) (s)$ A1

[3 marks]

c.ii.

Angle turned through is $0.1 \times 18 . 256^{2} =$ M1

$= 33.329 \dots$ A1

$\frac{33.329}{2 π}$ M1

$\frac{33.329}{2 π} = 5.30 \dots$

$5$ complete revolutions A1

[4 marks]

c.iii.

Examiners report

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

c.i.

[N/A]

c.ii.

[N/A]

c.iii.

A transformation, $T$ , of a plane is represented by $r' = P r + q$ , where $P$ is a $2 \times 2$ matrix, $q$ is a $2 \times 1$ vector, $r$ is the position vector of a point in the plane and $r'$ the position vector of its image under $T$ .

The triangle $OAB$ has coordinates $(0, 0)$ , $(0, 1)$ and $(1, 0)$ . Under T, these points are transformed to $(0, 1)$ , $(\frac{1}{4}, 1 + \frac{\sqrt{3}}{4})$ and $(\frac{\sqrt{3}}{4}, \frac{3}{4})$ respectively.

$P$ can be written as $P = R S$ , where $S$ and $R$ are matrices.

$S$ represents an enlargement with scale factor $0.5$ , centre $(0, 0)$ .

$R$ represents a rotation about $(0, 0)$ .

The transformation $T$ can also be described by an enlargement scale factor $\frac{1}{2}$ , centre $(a, b)$ , followed by a rotation about the same centre $(a, b)$ .

By considering the image of $(0, 0)$ , find $q$ .

[2]

a.i.

By considering the image of $(1, 0)$ and $(0, 1)$ , show that

$P = (\begin{matrix} \frac{\sqrt{3}}{4} & \frac{1}{4} \\ - \frac{1}{4} & \frac{\sqrt{3}}{4} \end{matrix})$ .

[4]

a.ii.

Write down the matrix $S$ .

[1]

Use $P = R S$ to find the matrix $R$ .

[4]

c.i.

Hence find the angle and direction of the rotation represented by $R$ .

[3]

c.ii.

Write down an equation satisfied by $(\begin{matrix} a \\ b \end{matrix})$ .

[1]

d.i.

Find the value of $a$ and the value of $b$ .

[3]

d.ii.

Markscheme

$P (\begin{matrix} 0 \\ 0 \end{matrix}) + q = (\begin{matrix} 0 \\ 1 \end{matrix})$ (M1)

$q = (\begin{matrix} 0 \\ 1 \end{matrix})$ A1

[2 marks]

a.i.

EITHER

$P (\begin{matrix} 1 \\ 0 \end{matrix}) + (\begin{matrix} 0 \\ 1 \end{matrix}) = (\begin{matrix} \frac{\sqrt{3}}{4} \\ \frac{3}{4} \end{matrix})$ M1

hence $P (\begin{matrix} 1 \\ 0 \end{matrix}) = (\begin{matrix} \frac{\sqrt{3}}{4} \\ - \frac{1}{4} \end{matrix})$ A1

$P (\begin{matrix} 0 \\ 1 \end{matrix}) + (\begin{matrix} 0 \\ 1 \end{matrix}) = (\begin{matrix} \frac{1}{4} \\ 1 + \frac{\sqrt{3}}{4} \end{matrix})$ M1

hence $P (\begin{matrix} 0 \\ 1 \end{matrix}) = (\begin{matrix} \frac{1}{4} \\ \frac{\sqrt{3}}{4} \end{matrix})$ A1

$(\begin{matrix} a & b \\ c & d \end{matrix}) (\begin{matrix} 1 \\ 0 \end{matrix}) + (\begin{matrix} 0 \\ 1 \end{matrix}) = (\begin{matrix} \frac{\sqrt{3}}{4} \\ \frac{3}{4} \end{matrix})$ M1

hence $(\begin{matrix} a & b \\ c & d \end{matrix}) (\begin{matrix} 1 \\ 0 \end{matrix}) = (\begin{matrix} \frac{\sqrt{3}}{4} \\ - \frac{1}{4} \end{matrix})$ A1

$(\begin{matrix} a \\ c \end{matrix}) = (\begin{matrix} \frac{\sqrt{3}}{4} \\ - \frac{1}{4} \end{matrix})$

$(\begin{matrix} a & b \\ c & d \end{matrix}) (\begin{matrix} 0 \\ 1 \end{matrix}) + (\begin{matrix} 0 \\ 1 \end{matrix}) = (\begin{matrix} \frac{1}{4} \\ 1 + \frac{\sqrt{3}}{4} \end{matrix})$ M1

$(\begin{matrix} a & b \\ c & d \end{matrix}) (\begin{matrix} 0 \\ 1 \end{matrix}) = (\begin{matrix} \frac{1}{4} \\ \frac{\sqrt{3}}{4} \end{matrix})$ A1

$(\begin{matrix} b \\ d \end{matrix}) = (\begin{matrix} \frac{1}{4} \\ \frac{\sqrt{3}}{4} \end{matrix})$

THEN

$\Rightarrow P = (\begin{matrix} \frac{\sqrt{3}}{4} & \frac{1}{4} \\ - \frac{1}{4} & \frac{\sqrt{3}}{4} \end{matrix})$ AG

[4 marks]

a.ii.

$(\begin{matrix} \frac{1}{2} & 0 \\ 0 & \frac{1}{2} \end{matrix})$ A1

[1 mark]

EITHER

$S^{- 1} = (\begin{matrix} 2 & 0 \\ 0 & 2 \end{matrix})$ (A1)

$R = P S^{- 1}$ (M1)

Note: The M1 is for an attempt at rearranging the matrix equation. Award even if the order of the product is reversed.

$R = (\begin{matrix} \frac{\sqrt{3}}{4} & \frac{1}{4} \\ - \frac{1}{4} & \frac{\sqrt{3}}{4} \end{matrix}) (\begin{matrix} 2 & 0 \\ 0 & 2 \end{matrix})$ (A1)

$(\begin{matrix} \frac{\sqrt{3}}{4} & \frac{1}{4} \\ - \frac{1}{4} & \frac{\sqrt{3}}{4} \end{matrix}) = R (\begin{matrix} 0.5 & 0 \\ 0 & 0.5 \end{matrix})$

let $R = (\begin{matrix} a & b \\ c & d \end{matrix})$

attempt to solve a system of equations M1

$\frac{\sqrt{3}}{4} = 0.5 a, \frac{1}{4} = 0.5 b$

$- \frac{1}{4} = 0.5 c, \frac{\sqrt{3}}{4} = 0.5 d$ A2

Note: Award A1 for two correct equations, A2 for all four equations correct.

THEN

$R = (\begin{matrix} \frac{\sqrt{3}}{2} & \frac{1}{2} \\ - \frac{1}{2} & \frac{\sqrt{3}}{2} \end{matrix})$ OR $(\begin{matrix} 0.866 & 0.5 \\ - 0.5 & 0.866 \end{matrix})$ OR $((\begin{matrix} 0.866025 \dots & 0.5 \\ - 0.5 & 0.866025 \dots \end{matrix}))$ A1

Note: The correct answer can be obtained from reversing the matrices, so do not award if incorrect product seen. If the given answer is obtained from the product $R = S^{- 1} P$ , award (A1)(M1)(A0)A0.

[4 marks]

c.i.

clockwise A1

arccosine or arcsine of value in matrix seen (M1)

$30 °$ A1

Note: Both A1 marks are dependent on the answer to part (c)(i) and should only be awarded for a valid rotation matrix.

[3 marks]

c.ii.

METHOD 1

$(\begin{matrix} a \\ b \end{matrix}) = P (\begin{matrix} a \\ b \end{matrix}) + q$ A1

METHOD 2

$(\begin{matrix} x' \\ y' \end{matrix}) = P (\begin{matrix} x - a \\ y - b \end{matrix}) + (\begin{matrix} a \\ b \end{matrix})$ A1

Note: Accept substitution of $x$ and $y$ (and $x'$ and $y'$ ) with particular points given in the question.

[1 mark]

d.i.

METHOD 1

solving $(\begin{matrix} a \\ b \end{matrix}) = P (\begin{matrix} a \\ b \end{matrix}) + q$ using simultaneous equations or $a = {(I - P)}^{- 1} q$ (M1)

$a = 0.651 (0.651084 \dots), b = 1.48 (1.47662 \dots)$ A1A1

$(a = \frac{5 + 2 \sqrt{3}}{13}, b = \frac{14 + 3 \sqrt{3}}{13})$

METHOD 2

$(\begin{matrix} 0 \\ 1 \end{matrix}) = P (\begin{matrix} 0 - a \\ 0 - b \end{matrix}) + (\begin{matrix} a \\ b \end{matrix})$ (M1)

Note: This line, with any of the points substituted, may be seen in part (d)(i) and if so the M1 can be awarded there.

$(\begin{matrix} 0 \\ 1 \end{matrix}) = (I - P) (\begin{matrix} a \\ b \end{matrix})$

$a = 0.651084 \dots, b = 1.47662 \dots$ A1A1

$(a = \frac{5 + 2 \sqrt{3}}{13}, b = \frac{14 + 3 \sqrt{3}}{13})$

[3 marks]

d.ii.

Examiners report

Part (i) proved to be straightforward for most candidates. A common error in part (ii) was for candidates to begin with the matrix P and to show it successfully transformed the points to their images. This received no marks. For a ‘show that’ question it is expected that the work moves to rather than from the given answer.

a.i.

[N/A]

a.ii.

(b), (c) These two parts dealt generally with more familiar aspects of matrix transformations and were well done.

c.i.

[N/A]

c.ii.

The trick of recognizing that $(a, b)$ was invariant was generally not seen and as such the question could not be successfully answered.

d.i.

[N/A]

d.ii.

A sector of a circle, centre $O$ and radius $4.5 m$ , is shown in the following diagram.

A square field with side $8 m$ has a goat tied to a post in the centre by a rope such that the goat can reach all parts of the field up to $4.5 m$ from the post.

^{[Source: mynamepong, n.d. Goat [image online] Available at: https://thenounproject.com/term/goat/1761571/}
^{This file is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported (CC BY-SA 3.0)}
^{https://creativecommons.org/licenses/by-sa/3.0/deed.en [Accessed 22 April 2010] Source adapted.]}

Let $V$ be the volume of grass eaten by the goat, in cubic metres, and $t$ be the length of time, in hours, that the goat has been in the field.

The goat eats grass at the rate of $\frac{d V}{d t} = 0.3 t e^{- t}$ .

Find the angle $AÔB$ .

[3]

a.i.

Find the area of the shaded segment.

[5]

a.ii.

Find the area of the field that can be reached by the goat.

[4]

Find the value of $t$ at which the goat is eating grass at the greatest rate.

[2]

The goat is tied in the field for $8$ hours.

Find the total volume of grass eaten by the goat during this time.

[3]

Markscheme

$(\frac{1}{2} AÔB =) arccos (\frac{4}{4.5}) = 27.266 \dots$ (M1)(A1)

$AÔB = 54.532 \dots \approx 54.5 °$ ( $0.951764 \dots \approx 0.952$ radians) A1

Note: Other methods may be seen; award (M1)(A1) for use of a correct trigonometric method to find an appropriate angle and then A1 for the correct answer.

[3 marks]

a.i.

finding area of triangle

EITHER

area of triangle $= \frac{1}{2} \times 4 . 5^{2} \times \sin (54.532 \dots)$ (M1)

Note: Award M1 for correct substitution into formula.

$= 8.24621 \dots \approx 8.25 m^{2}$ (A1)

$AB = 2 \times \sqrt{4 . 5^{2} - 4^{2}} = 4.1231 \dots$ (M1)

area triangle $= \frac{4.1231 \dots \times 4}{2}$

$= 8.24621 \dots \approx 8.25 m^{2}$ (A1)

finding area of sector

EITHER

area of sector $= \frac{54.532 \dots}{360} \times π \times 4 . 5^{2}$ (M1)

$= 9.63661 \dots \approx 9.64 m^{2}$ (A1)

area of sector $= \frac{1}{2} \times 0.9517641 \dots \times 4 . 5^{2}$ (M1)

$= 9.63661 \dots \approx 9.64 m^{2}$ (A1)

THEN

area of segment $= 9.63661 \dots - 8.24621 \dots$

$= 1.39 m^{2} (1.39040 \dots)$ A1

[5 marks]

a.ii.

METHOD 1

$π \times 4 . 5^{2} (63.6172 \dots)$ (A1)

$4 \times 1.39040 . . . (5.56160)$ (A1)

subtraction of four segments from area of circle (M1)

$= 58.1 m^{2} (58.055 \dots)$ A1

METHOD 2

angle of sector $= 90 - 54.532 \dots (\frac{π}{2} - 0.951764 \dots)$ (A1)

area of sector $= \frac{90 - 54.532 \dots}{360} \times π \times 4 . 5^{2} (= 6.26771 \dots)$ (A1)

area is made up of four triangles and four sectors (M1)

total area $= (4 \times 8.2462 \dots) + (4 \times 6.26771 \dots)$

$= 58.1 m^{2} (58.055 \dots)$ A1

[4 marks]

sketch of $\frac{d V}{d t}$ OR $\frac{d V}{d t} = 0.110363 \dots$ OR attempt to find where $\frac{d^{2} V}{d t^{2}} = 0$ (M1)

$t = 1$ hour A1

[2 marks]

recognizing $V = \int \frac{d V}{d t} d t$ (M1)

$\int_{0}^{8} 0.3 t e^{- 1} d t$ (A1)

volume eaten is $0.299 \dots m^{3} (0.299094 \dots)$ A1

[3 marks]

Examiners report

Generally, this question was answered well but provided a good example of final marks being lost due to premature rounding. Some candidates gave a correct three significant figure intermediate answer of 27.3˚ for the angle in the right-angles triangle and then doubled it to get 54.6˚ as a final answer. This did not receive the final answer mark as the correct answer is 54.5˚ to three significant figures. Premature rounding needs to be avoided in all questions.

a.i.

[N/A]

a.ii.

Unfortunately, many candidates failed to see the connection to part (a). Indeed, the most common answer was to assume the goat could eat all the grass in a circle of radius 4.5m.

Most candidates completed this question successfully by graphing the function. A few tried to differentiate the function again and, in some cases, also managed to obtain the correct answer.

This was a question that was pleasingly answered correctly by many candidates who recognized that integration was needed to find the answer. As in part (c) a few tried to do the integration ‘by hand’, and were largely unsuccessful.

Consider the curve $y = \sqrt{x}$ .

The shape of a piece of metal can be modelled by the region bounded by the functions $f$ , $g$ , the $x$ -axis and the line segment $[AB]$ , as shown in the following diagram. The units on the $x$ and $y$ axes are measured in metres.

The piecewise function $f$ is defined by

$f (x) = {\begin{matrix} \sqrt{x} & 0 \leq x \leq 0.16 \\ 1.25 x + 0.2 & 0.16 < x \leq 0.5 \end{matrix}$

The graph of $g$ is obtained from the graph of $f$ by:

a stretch scale factor of $\frac{1}{2}$ in the $x$ direction,
followed by a stretch scale factor $\frac{1}{2}$ in the $y$ direction,
followed by a translation of $0.2$ units to the right.

Point $A$ lies on the graph of $f$ and has coordinates $(0.5, 0.825)$ . Point $B$ is the image of $A$ under the given transformations and has coordinates $(p, q)$ .

The piecewise function $g$ is given by

$g (x) = {\begin{matrix} h (x) & 0.2 \leq x \leq a \\ 1.25 x + b & a < x \leq p \end{matrix}$

The area enclosed by $y = g (x)$ , the $x$ -axis and the line $x = p$ is $0.0627292 m^{2}$ correct to six significant figures.

Find $\frac{d y}{d x}$ .

[2]

a.i.

Hence show that the equation of the tangent to the curve at the point $(0.16, 0.4)$ is $y = 1.25 x + 0.2$ .

[2]

a.ii.

Find the value of $p$ and the value of $q$ .

[2]

Find an expression for $h (x)$ .

[2]

c.i.

Find the value of $a$ .

[1]

c.ii.

Find the value of $b$ .

[2]

c.iii.

Find the area enclosed by $y = f (x)$ , the $x$ -axis and the line $x = 0.5$ .

[3]

d.i.

Find the area of the shaded region on the diagram.

[4]

d.ii.

Markscheme

$y = x^{\frac{1}{2}}$ (M1)

$\frac{d y}{d x} = \frac{1}{2} x^{- \frac{1}{2}}$ A1

[2 marks]

a.i.

gradient at $x = 0.16$ is $\frac{1}{2} \times \frac{1}{\sqrt{0.16}}$ M1

$= 1.25$

EITHER

$y - 0.4 = 1.25 (x - 0.16)$ M1

$0.4 = 1.25 (0.16) + b$ M1

Note: Do not allow working backwards from the given answer.

THEN

hence $y = 1.25 x + 0.2$ AG

[2 marks]

a.ii.

$p = 0.45, q = 0.4125$ (or $0.413$ ) (accept " $(0.45, 0.4125)$ ") A1A1

[2 marks]

$(h (x) =) \frac{1}{2} \sqrt{2 (x - 0.2)}$ A2

Note: Award A1 if only two correct transformations are seen.

[2 marks]

c.i.

$(a =) 0.28$ A1

[1 mark]

c.ii.

EITHER

Correct substitution of their part (b) (or $(0.28, 0.2)$ ) into the given expression (M1)

$\frac{1}{2} (1.25 \times 2 (x - 0.2) + 0.2)$ (M1)

Note: Award M1 for transforming the equivalent expression for $f$ correctly.

THEN

$(b =) - 0.15$ A1

[2 marks]

c.iii.

recognizing need to add two integrals (M1)

$\int_{0}^{0.16} \sqrt{x} d x + \int_{0.16}^{0.5} (1.25 x + 0.2) d x$ (A1)

Note: The second integral could be replaced by the formula for the area of a trapezoid $\frac{1}{2} \times 0.34 (0.4 + 0.825)$ .

$0.251 m^{2} (0.250916 \dots)$ A1

[3 marks]

d.i.

EITHER

area of a trapezoid $\frac{1}{2} \times 0.05 (0.4125 + 0.825) = 0.0309375$ (M1)(A1)

$\int_{0.45}^{0.5} (8.25 x - 3.3) d x = 0.0309375$ (M1)(A1)

Note: If the rounded answer of $0.413$ from part (b) is used, the integral is $\int_{0.45}^{0.5} (8.24 x - 3.295) d x = 0.03095$ which would be awarded (M1)(A1).

THEN

shaded area $= 0.250916 \dots - 0.0627292 - 0.0309375$ (M1)

Note: Award (M1) for the subtraction of both $0.0627292 \dots$ and their area for the trapezoid from their answer to (a)(i).

$= 0.157 m^{2} (0.15725)$ A1

[4 marks]

d.ii.

Examiners report

The differentiation using the power rule was well done. In part (ii) some candidates felt it was sufficient to refer to the equation being the same as the one generated by their calculator. Generally, for ‘show that’ questions an algebraic derivation is expected.

a.i.

[N/A]

a.ii.

The candidates were successful at applying transformations to points but very few were able to apply these transformations to derive the correct function h. In most cases it was due to not appreciating the effect the horizontal transformations have on x.

c.i.

[N/A]

c.ii.

[N/A]

c.iii.

Part (i) was frequently done well using the inbuilt functionality of the GDC. Part (ii) was less structured, and candidates needed to create a clear diagram so they could easily see which areas needed to be subtracted. Most of those who were successful used the formula for the trapezoid for the area they needed to find, though others were also successful through finding the equation of the line AB.

d.i.

[N/A]

d.ii.

The cost adjacency matrix for the complete graph K₆ is given below.

It represents the distances in kilometres along dusty tracks connecting villages on an island. Find the minimum spanning tree for this graph; in all 3 cases state the order in which the edges are added.

It is desired to tarmac some of these tracks so that it is possible to walk from any village to any other village walking entirely on tarmac.

Briefly explain the two differences in the application of Prim’s and Kruskal’s algorithms for finding a minimum spanning tree in a weighted connected graph.

[2]

Using Kruskal’s algorithm.

[2]

b.i.

Using Prim’s algorithm starting at vertex A.

[2]

b.ii.

Using Prim’s algorithm starting at vertex F.

[2]

b.iii.

State the total minimum length of the tracks that have to be tarmacked.

[2]

c.i.

Sketch the tracks that are to be tarmacked.

[2]

c.ii.

Markscheme

In Prim’s algorithm you start at a particular (given) vertex, whereas in Kruskal’s you start with the smallest edge. A1

In Prim’s as smallest edges are added (never creating a circuit) the created graph always remains connected, whereas in Kruskal’s this requirement to always be connected is not necessary. A1

[2 marks]

Edges added in the order

AB EF AC AD AE A1A1

[note A1 for the first 2 edges A1 for other 3]

[2 marks]

b.i.

Edges added in the order

AB AC AD AE EF A1A1

[note A1 for the first 2 edges A1 for other 3]

[2 marks]

b.ii.

Edges added in the order

FE AE AB AC AD A1A1

[note A1 for the first 2 edges A1 for other 3]

[2 marks]

b.iii.

$1 + 2 + 3 + 4 + 5 = 15$ M1A1

[2 marks]

c.i.

[2 marks]

c.ii.

Examiners report

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

b.iii.

[N/A]

c.i.

[N/A]

c.ii.

At an archery tournament, a particular competition sees a ball launched into the air while an archer attempts to hit it with an arrow.

The path of the ball is modelled by the equation

$(\begin{matrix} x \\ y \end{matrix}) = (\begin{matrix} 5 \\ 0 \end{matrix}) + t (\begin{matrix} u_{x} \\ u_{y} - 5 t \end{matrix})$

where $x$ is the horizontal displacement from the archer and $y$ is the vertical displacement from the ground, both measured in metres, and $t$ is the time, in seconds, since the ball was launched.

$u_{x}$ is the horizontal component of the initial velocity
$u_{y}$ is the vertical component of the initial velocity.

In this question both the ball and the arrow are modelled as single points. The ball is launched with an initial velocity such that $u_{x} = 8$ and $u_{y} = 10$ .

An archer releases an arrow from the point $(0, 2)$ . The arrow is modelled as travelling in a straight line, in the same plane as the ball, with speed $60 {m s}^{- 1}$ and an angle of elevation of $10 °$ .

Find the initial speed of the ball.

[2]

a.i.

Find the angle of elevation of the ball as it is launched.

[2]

a.ii.

Find the maximum height reached by the ball.

[3]

Assuming that the ground is horizontal and the ball is not hit by the arrow, find the $x$ coordinate of the point where the ball lands.

[3]

For the path of the ball, find an expression for $y$ in terms of $x$ .

[3]

Determine the two positions where the path of the arrow intersects the path of the ball.

[4]

Determine the time when the arrow should be released to hit the ball before the ball reaches its maximum height.

[4]

Markscheme

$\sqrt{10^{2} + 8^{2}}$ (M1)

$= 12.8 (12.8062 \dots, \sqrt{164}) (m s^{- 1})$ A1

[2 marks]

a.i.

$\tan^{- 1} (\frac{10}{8})$ (M1)

$= 0.896$ OR $51.3$ ( $0.896055 \dots$ OR $51.3401 \dots °$ ) A1

Note: Accept $0.897$ or $51.4$ from use of $arcsin (\frac{10}{12.8})$ .

[2 marks]

a.ii.

$y = t (10 - 5 t)$ (M1)

Note: The M1 might be implied by a correct graph or use of the correct equation.

METHOD 1 – graphical Method

sketch graph (M1)

Note: The M1 might be implied by correct graph or correct maximum (eg $t = 1$ ).

max occurs when $y = 5 m$ A1

METHOD 2 – calculus

differentiating and equating to zero (M1)

$\frac{d y}{d t} = 10 - 10 t = 0$

$t = 1$

$y (= 1 (10 - 5)) = 5 m$ A1

METHOD 3 – symmetry

line of symmetry is $t = 1$ (M1)

$y (= 1 (10 - 5)) = 5 m$ A1

[3 marks]

attempt to solve $t (10 - 5 t) = 0$ (M1)

$t = 2$ (or $t = 0$ ) (A1)

$x (= 5 + 8 \times 2) = 21 m$ A1

Note: Do not award the final A1 if $x = 5$ is also seen.

[3 marks]

METHOD 1

$t = \frac{x - 5}{8}$ M1A1

$y = (\frac{x - 5}{8}) (10 - 5 \times \frac{x - 5}{8})$ A1

METHOD 2

$y = k (x - 5) (x - 21)$ A1

when $x = 13, y = 5$ so $k = \frac{5}{(13 - 5) (13 - 21)} = - \frac{5}{64}$ M1A1

$(y = - \frac{5}{64} (x - 5) (x - 21))$

METHOD 3

if $y = a x^{2} + b x + c$

$0 = 25 a + 5 b + c$
$5 = 169 a + 13 b + c$
$0 = 441 a + 21 b + c$ M1A1

solving simultaneously, $a = - \frac{5}{64}, b = \frac{130}{64}, c = - \frac{525}{64}$ A1

( $y = - \frac{5}{64} x^{2} + \frac{130}{64} x - \frac{525}{64}$ )

METHOD 4

use quadratic regression on $(5, 0), (13, 5), (21, 0)$ M1A1

$y = - \frac{5}{64} x^{2} + \frac{130}{64} x - \frac{525}{64}$ A1

Note: Question asks for expression; condone omission of " $y =$ ".

[3 marks]

trajectory of arrow is $y = x \tan 10 + 2$ (A1)

intersecting $y = x \tan 10 + 2$ and their answer to (d) (M1)

$(8.66, 3.53) ((8.65705 \dots, 3.52647 \dots))$ A1

$(15.1, 4.66) ((15.0859 \dots, 4.66006 \dots))$ A1

[4 marks]

when $x_{target} = 8.65705 \dots, t_{target} = \frac{8.65705 \dots - 5}{8} = 0.457132 \dots s$ (A1)

attempt to find the distance from point of release to intersection (M1)

$\sqrt{8.65705 \dots^{2} + {(3.52647 \dots - 2)}^{2}} (= 8.79060 \dots m)$

time for arrow to get there is $\frac{8.79060 \dots}{60} = 0.146510 \dots s$ (A1)

so the arrow should be released when

$t = 0.311 (s) (0.310622 \dots (s))$ A1

[4 marks]

Examiners report

This question was found to be the most difficult on the paper. There were a good number of good solutions to parts (a) and part (b), frequently with answers just written down with no working. Part (c) caused some difficulties with confusing variables. The most significant difficulties started with part (d) and became greater to the end of the question. Few candidates were able to work through the final two parts.

a.i.

[N/A]

a.ii.

[N/A]

The matrices A and B are defined by $A = \left( {\begin{array}{*{20}{c}} 3&{ - 2} \\ 2&4 \end{array}} \right)$ and $B = \left( {\begin{array}{*{20}{c}} { - 1}&0 \\ 0&1 \end{array}} \right)$ .

Triangle X is mapped onto triangle Y by the transformation represented by AB. The coordinates of triangle Y are (0, 0), (−30, −20) and (−16, 32).

Describe fully the geometrical transformation represented by B.

[2]

Find the coordinates of triangle X.

[5]

Find the area of triangle X.

[2]

c.i.

Hence find the area of triangle Y.

[3]

c.ii.

Matrix A represents a combination of transformations:

A stretch, with scale factor 3 and y-axis invariant;
Followed by a stretch, with scale factor 4 and x-axis invariant;
Followed by a transformation represented by matrix C.

Find matrix C.

[4]

Markscheme

reflection in the y-axis A1A1

[2 marks]

$X = {\left( {AB} \right)^{ - 1}}Y$ M1

EITHER

$AB = \left( {\begin{array}{*{20}{c}} { - 3}&{ - 2} \\ { - 2}&4 \end{array}} \right)$ , so ${\left( {AB} \right)^{ - 1}} = \left( {\begin{array}{*{20}{c}} { - \frac{1}{4}}&{ - \frac{1}{8}} \\ { - \frac{1}{8}}&{\frac{3}{{16}}} \end{array}} \right)$ M1A1

$X = {B^{ - 1}}{A^{ - 1}}Y$ M1A1

THEN

$X = \left( {\begin{array}{*{20}{c}} 0&{10}&0 \\ 0&0&8 \end{array}} \right)$ (A1)

So the coordinates are (0, 0), (10, 0) and (0, 8). A1

[5 marks]

$\frac{{10 \times 8}}{2} = 40$ units² M1A1

[2 marks]

c.i.

$\det \left( {AB} \right) = - 16$ M1A1

Area $= 40 \times 16 = 640$ units² A1

[3 marks]

c.ii.

A stretch, with scale factor 3 and y-axis invariant is given by $\left( {\begin{array}{*{20}{c}} 3&0 \\ 0&1 \end{array}} \right)$ A1

A stretch, with scale factor 4 and x-axis invariant is given by $\left( {\begin{array}{*{20}{c}} 1&0 \\ 0&4 \end{array}} \right)$ A1

So $C = A{\left( {\begin{array}{*{20}{c}} 3&0 \\ 0&1 \end{array}} \right)^{ - 1}}{\left( {\begin{array}{*{20}{c}} 1&0 \\ 0&4 \end{array}} \right)^{ - 1}} = \left( {\begin{array}{*{20}{c}} 1&{ - \frac{1}{2}} \\ {\frac{2}{3}}&1 \end{array}} \right)$ M1A1

[4 marks]

Examiners report

[N/A]

c.i.

[N/A]

c.ii.

[N/A]

Let G be the graph below.

Find the total number of Hamiltonian cycles in G, starting at vertex A. Explain your answer.

[3]

Find a minimum spanning tree for the subgraph obtained by deleting A from G.

[3]

b.i.

Hence, find a lower bound for the travelling salesman problem for G.

[3]

b.ii.

Give an upper bound for the travelling salesman problem for the graph above.

[2]

Show that the lower bound you have obtained is not the best possible for the solution to the travelling salesman problem for G.

[3]

Markscheme

Starting from vertex A there are 4 choices. From the next vertex there are three choices, etc… M1R1

So the number of Hamiltonian cycles is 4! = 24. A1 N1

[3 marks]

Start (for instance) at B, using Prim′s algorithm Then D is the nearest vertex M1

Next E is the nearest vertex A1

Finally C is the nearest vertex So a minimum spanning tree is B → D → E → C A1 N1

[3 marks]

b.i.

A lower bound for the travelling salesman problem is then obtained by adding the weights of AB and AE to the weight of the minimum M1

spanning tree (ie 20) A1

A lower bound is then 20 + 7 + 6 = 33 A1 N1

[3 marks]

b.ii.

ABCDE is an Hamiltonian cycle A1

Thus an upper bound is given by 7 + 9 + 9 + 8 + 6 = 39 A1

[2 marks]

Eliminating C from G a minimum spanning tree is E → A → B → D M1

of weight 18 A1

Adding BC to CE(18 + 9 + 7) gives a lower bound of 34 > 33 A1

So 33 not the best lower bound. AG N0

[3 marks]

Examiners report

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

The matrix A is defined by $A = \left( {\begin{array}{*{20}{c}} 3&0 \\ 0&2 \end{array}} \right)$ .

Pentagon, P, which has an area of 7 cm², is transformed by A.

The matrix B is defined by $B = \frac{1}{2}\left( {\begin{array}{*{20}{c}} {3\sqrt 3 }&3 \\ { - 2}&{2\sqrt 3 } \end{array}} \right)$ .

B represents the combined effect of the transformation represented by a matrix X, followed by the transformation represented by A.

Describe fully the geometrical transformation represented by A.

[5]

Find the area of the image of P.

[2]

Find the matrix X.

[3]

Describe fully the geometrical transformation represented by X.

[2]

Markscheme

stretch A1

scale factor 3, A1

y-axis invariant (condone parallel to the x-axis) A1

and

stretch, scale factor 2, A1

x-axis invariant (condone parallel to the y-axis) A1

[5 marks]

${\text{det}}\left( A \right) = 6$ A1

$7 \times 6 = 42\,{\text{c}}{{\text{m}}^2}$ A1

[2 marks]

$B = AX$ (A1)

$X = {A^{ - 1}}B$ (M1)

$X = \left( {\begin{array}{*{20}{c}} {0.866}&{0.5} \\ { - 0.5}&{0.866} \end{array}} \right)\left( { = \left( {\begin{array}{*{20}{c}} {\frac{{\sqrt 3 }}{2}}&{\frac{1}{2}} \\ { - \frac{1}{2}}&{\frac{{\sqrt 3 }}{2}} \end{array}} \right)} \right)$ A1

[3 marks]

Rotation A1

clockwise by 30° about the origin A1

[2 marks]

Examiners report

[N/A]

In triangle ${\text{PQR, PR}} = 12{\text{ cm, QR}} = p{\text{ cm, PQ}} = r{\text{ cm}}$ and ${\rm{Q\hat PR}} = 30^\circ$ .

Consider the possible triangles with ${\text{QR}} = 8{\text{ cm}}$ .

Consider the case where $p$ , the length of QR is not fixed at 8 cm.

Use the cosine rule to show that ${r^2} - 12\sqrt 3 r + 144 - {p^2} = 0$ .

[2]

Calculate the two corresponding values of PQ.

[3]

Hence, find the area of the smaller triangle.

[3]

Determine the range of values of $p$ for which it is possible to form two triangles.

[7]

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

${p^2} = {12^2} + {r^2} - 2 \times 12 \times r \times \cos (30^\circ )$ M1A1

${r^2} - 12\sqrt 3 r + 144 - {p^2} = 0$ AG

[2 marks]

EITHER

${r^2} - 12\sqrt 3 r + 80 = 0$ (M1)

using the sine rule (M1)

THEN

${\text{PQ}} = 5.10{\text{ }}({\text{cm}})$ or A1

${\text{PQ}} = 15.7{\text{ }}({\text{cm}})$ A1

[3 marks]

${\text{area}} = \frac{1}{2} \times 12 \times 5.1008 \ldots \times \sin (30^\circ )$ M1A1

$= 15.3{\text{ }}({\text{c}}{{\text{m}}^2})$ A1

[3 marks]

METHOD 1

EITHER

${r^2} - 12\sqrt 3 r + 144 - {p^2} = 0$

discriminant $= {\left( {12\sqrt 3 } \right)^2} - 4 \times (144 - {p^2})$ M1

$= 4({p^2} - 36)$ A1

$({p^2} - 36) > 0$ M1

$p > 6$ A1

construction of a right angle triangle (M1)

$12\sin 30^\circ = 6$ M1(A1)

hence for two triangles $p > 6$ R1

THEN

$p < 12$ A1

$144 - {p^2} > 0$ to ensure two positive solutions or valid geometric argument R1

$\therefore 6 < p < 12$ A1

METHOD 2

diagram showing two triangles (M1)

$12\sin 30^\circ = 6$ M1A1

one right angled triangle when $p = 6$ (A1)

$\therefore p > 6$ for two triangles R1

$p < 12$ for two triangles A1

$6 < p < 12$ A1

[7 marks]

Examiners report

[N/A]

Find the set of values of $k$ that satisfy the inequality ${k^2} - k - 12 < 0$ .

[2]

The triangle ABC is shown in the following diagram. Given that $\cos B < \frac{1}{4}$ , find the range of possible values for AB.

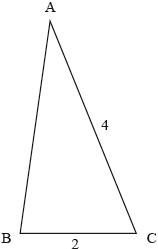
M17/5/MATHL/HP2/ENG/TZ2/04.b

[4]

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

${k^2} - k - 12 < 0$

$(k - 4)(k + 3) < 0$ (M1)

$- 3 < k < 4$ A1

[2 marks]

$\cos B = \frac{{{2^2} + {c^2} - {4^2}}}{{4c}}{\text{ }}({\text{or }}16 = {2^2} + {c^2} - 4c\cos B)$ M1

$\Rightarrow \frac{{{c^2} - 12}}{{4c}} < \frac{1}{4}$ A1

$\Rightarrow {c^2} - c - 12 < 0$

from result in (a)

$0 < {\text{AB}} < 4$ or $- 3 < {\text{AB}} < 4$ (A1)

but AB must be at least 2

$\Rightarrow 2 < {\text{AB}} < 4$ A1

Note: Allow $\leqslant {\text{AB}}$ for either of the final two A marks.

[4 marks]

Examiners report

[N/A]

A water trough which is 10 metres long has a uniform cross-section in the shape of a semicircle with radius 0.5 metres. It is partly filled with water as shown in the following diagram of the cross-section. The centre of the circle is O and the angle KOL is $\theta$ radians.

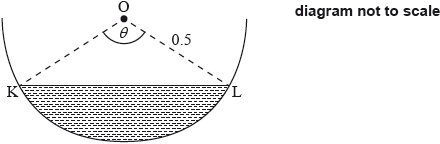
M17/5/MATHL/HP2/ENG/TZ1/08

The volume of water is increasing at a constant rate of $0.0008{\text{ }}{{\text{m}}^3}{{\text{s}}^{ - 1}}$ .

Find an expression for the volume of water $V{\text{ }}({{\text{m}}^3})$ in the trough in terms of $\theta$ .

[3]

Calculate $\frac{{{\text{d}}\theta }}{{{\text{d}}t}}$ when $\theta = \frac{\pi }{3}$ .

[4]

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

area of segment $= \frac{1}{2} \times {0.5^2} \times (\theta - \sin \theta )$ M1A1

$V = {\text{area of segment}} \times 10$

$V = \frac{5}{4}(\theta - \sin \theta )$ A1

[3 marks]

METHOD 1

$\frac{{{\text{d}}V}}{{{\text{d}}t}} = \frac{5}{4}(1 - \cos \theta )\frac{{{\text{d}}\theta }}{{{\text{d}}t}}$ M1A1

$0.0008 = \frac{5}{4}\left( {1 - \cos \frac{\pi }{3}} \right)\frac{{{\text{d}}\theta }}{{{\text{d}}t}}$ (M1)

$\frac{{{\text{d}}\theta }}{{{\text{d}}t}} = 0.00128{\text{ }}({\text{rad}}\,{s^{ - 1}})$ A1

METHOD 2

$\frac{{{\text{d}}\theta }}{{{\text{d}}t}} = \frac{{{\text{d}}\theta }}{{{\text{d}}V}} \times \frac{{{\text{d}}V}}{{{\text{d}}t}}$ (M1)

$\frac{{{\text{d}}V}}{{{\text{d}}\theta }} = \frac{5}{4}(1 - \cos \theta )$ A1

$\frac{{{\text{d}}\theta }}{{{\text{d}}t}} = \frac{{4 \times 0.0008}}{{5\left( {1 - \cos \frac{\pi }{3}} \right)}}$ (M1)

$\frac{{{\text{d}}\theta }}{{{\text{d}}t}} = 0.00128\left( {\frac{4}{{3125}}} \right)({\text{rad }}{s^{ - 1}})$ A1

[4 marks]

Examiners report

[N/A]

An aircraft’s position is given by the coordinates ( $x$ , $y$ , $z$ ), where $x$ and $y$ are the aircraft’s displacement east and north of an airport, and $z$ is the height of the aircraft above the ground. All displacements are given in kilometres.

The velocity of the aircraft is given as $\left( {\begin{array}{*{20}{c}} { - 150} \\ { - 50} \\ { - 20} \end{array}} \right)\,{\text{km}}\,{{\text{h}}^{ - 1}}$ .

At 13:00 it is detected at a position 30 km east and 10 km north of the airport, and at a height of 5 km. Let $t$ be the length of time in hours from 13:00.

If the aircraft continued to fly with the velocity given

When the aircraft is 4 km above the ground it continues to fly on the same bearing but adjusts the angle of its descent so that it will land at the point (0, 0, 0).

Write down a vector equation for the displacement, r of the aircraft in terms of $t$ .

[2]

verify that it would pass directly over the airport.

[2]

b.i.

state the height of the aircraft at this point.

[1]

b.ii.

find the time at which it would fly directly over the airport.

[1]

b.iii.

Find the time at which the aircraft is 4 km above the ground.

[2]

c.i.

Find the direct distance of the aircraft from the airport at this point.

[3]

c.ii.

Given that the velocity of the aircraft, after the adjustment of the angle of descent, is $\left( {\begin{array}{*{20}{c}} { - 150} \\ { - 50} \\ a \end{array}} \right){\text{km}}\,{{\text{h}}^{ - 1}}$ , find the value of $a$ .

[3]

Markscheme

r $= \left( {\begin{array}{*{20}{c}} {30} \\ {10} \\ 5 \end{array}} \right) + t\left( {\begin{array}{*{20}{c}} { - 150} \\ { - 50} \\ { - 20} \end{array}} \right)\,$ A1A1

[2 marks]

when $x = 0$ , $t = \frac{{30}}{{150}} = 0.2$ M1

EITHER

when $y = 0$ , $t = \frac{{10}}{{150}} = 0.2$ A1

since the two values of $t$ are equal the aircraft passes directly over the airport

$t = 0.2$ , $y = 0$ A1

[2 marks]

b.i.

height = 5 − 0.2 × 20 = 1 km A1

[1 mark]

b.ii.

time 13:12 A1

[1 mark]

b.iii.

$5 - 20t = 4 \Rightarrow t = \frac{1}{{20}}$ (3 minutes) (M1)

time 13:03 A1

[2 marks]

c.i.

displacement is $\left( {\begin{array}{*{20}{c}} {22.5} \\ {7.5} \\ 4 \end{array}} \right)$ A1

distance is $\sqrt {{{22.5}^2} + {{7.5}^2} + {4^2}}$ (M1)

= 24.1 km A1

[3 marks]

c.ii.

METHOD 1

time until landing is $12 - 3 = 9$ minutes M1

height to descend = $4\,{\text{km}}$

$a = \frac{{ - 4}}{{\frac{9}{{60}}}}$ M1

$= - 26.7$ A1

METHOD 2

$\left( {\begin{array}{*{20}{c}} { - 150} \\ { - 50} \\ a \end{array}} \right) = s\left( {\begin{array}{*{20}{c}} {22.5} \\ {7.5} \\ 4 \end{array}} \right)$ M1

$- 150 = 22.5\,s \Rightarrow s = - \frac{{20}}{3}$ M1

$a = - \frac{{20}}{3} \times 4$

$= - 26.7$ A1

[3 marks]

Examiners report

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

b.iii.

[N/A]

c.i.

[N/A]

c.ii.

[N/A]

Two submarines A and B have their routes planned so that their positions at time t hours, 0 ≤ t < 20 , would be defined by the position vectors r_A $= \left( \begin{gathered} \,2 \hfill \\ \,4 \hfill \\ - 1 \hfill \\ \end{gathered} \right) + t\left( \begin{gathered} - 1 \hfill \\ \,1 \hfill \\ - 0.15 \hfill \\ \end{gathered} \right)$ and r_B $= \left( \begin{gathered} \,0 \hfill \\ \,3.2 \hfill \\ - 2 \hfill \\ \end{gathered} \right) + t\left( \begin{gathered} - 0.5 \hfill \\ \,1.2 \hfill \\ \,0.1 \hfill \\ \end{gathered} \right)$ relative to a fixed point on the surface of the ocean (all lengths are in kilometres).

To avoid the collision submarine B adjusts its velocity so that its position vector is now given by

r_B $= \left( \begin{gathered} \,0 \hfill \\ \,3.2 \hfill \\ - 2 \hfill \\ \end{gathered} \right) + t\left( \begin{gathered} - 0.45 \hfill \\ \,1.08 \hfill \\ \,0.09 \hfill \\ \end{gathered} \right)$ .

Show that the two submarines would collide at a point P and write down the coordinates of P.

[4]

Find the value of t when submarine B passes through P.

[2]

b.ii.

Find the value of t when the two submarines are closest together.

[2]

c.ii.

Find the distance between the two submarines at this time.

[1]

c.iii.

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

r_A= r_{B (M1)}

2 − t = − 0.5t ⇒ t = 4 A1

checking t = 4 satisfies 4 + t = 3.2 + 1.2t and − 1 − 0.15t = − 2 + 0.1t R1

P(−2, 8, −1.6) A1

Note: Do not award final A1 if answer given as column vector.

[4 marks]

$\left( \begin{gathered} \, - 0.45t \hfill \\ 3.2 + 1.08t \hfill \\ - 2 + 0.09t \hfill \\ \end{gathered} \right) = \left( \begin{gathered} - 2 \hfill \\ \,8 \hfill \\ - 1.6 \hfill \\ \end{gathered} \right)$ M1

Note: The M1 can be awarded for any one of the resultant equations.

$\Rightarrow t = \frac{{40}}{9} = 4.44 \ldots$ A1

[2 marks]

b.ii.

minimum when $\frac{{{\text{d}}D}}{{{\text{d}}t}} = 0$ (M1)

t = 3.83 A1

[2 marks]

c.ii.

0.511 (km) A1

[1 mark]

c.iii.

Examiners report

[N/A]

b.ii.

[N/A]

c.ii.

[N/A]

c.iii.

A canal system divides a city into six land masses connected by fifteen bridges, as shown in the diagram below.

State with reasons whether or not this graph has

Draw a graph to represent this map.

[2]

Write down the adjacency matrix of the graph.

[2]

List the degrees of each of the vertices.

[2]

an Eulerian circuit.

[2]

d.i.

an Eulerian trail.

[2]

d.ii.

Find the number of walks of length 4 from E to F.

[2]

Markscheme

[2 marks]

M = $\begin{array}{*{20}{c}} {} \\ {\text{A}} \\ {\text{B}} \\ {\text{C}} \\ {\text{D}} \\ {\text{E}} \\ {\text{F}} \end{array}\begin{array}{*{20}{c}} {\begin{array}{*{20}{c}} {\text{A}}&{\text{B}}&{\text{C}}&{\text{D}}&{\text{E}}&{\text{F}} \end{array}} \\ {\left( {\begin{array}{*{20}{c}} 0&1&2&1&2&2 \\ 1&0&0&0&1&2 \\ 2&0&0&1&0&1 \\ 1&0&1&0&1&0 \\ 2&1&0&1&0&1 \\ 2&2&1&0&1&0 \end{array}} \right)} \end{array}$ A2

Note: Award A1 for one error or omission, A0 for more than one error or omission. Two symmetrical errors count as one error.

[2 marks]

A B C D E F

(8, 4 4, 3 5, 6) A2

Note: Award no more than A1 for one error, A0 for more than one error.

[2 marks]

no, because there are odd vertices M1A1

[2 marks]

d.i.

yes, because there are exactly two odd vertices M1A1

[2 marks]

d.ii.

M⁴ = $\begin{array}{*{20}{c}} {} \\ {\text{A}} \\ {\text{B}} \\ {\text{C}} \\ {\text{D}} \\ {\text{E}} \\ {\text{F}} \end{array}\begin{array}{*{20}{c}} {\begin{array}{*{20}{c}} {\text{A}}&{}&{\text{B}}&{}&{\text{C}}&{}&{\text{D}}&{}&{\text{E}}&{}&{\text{F}} \end{array}} \\ {\left( {\begin{array}{*{20}{c}} {309\,}&{174}&{140}&{118}&{170}&{214} \\ {174}&{117}&{106}&{70}&{122}&{132} \\ {140\,}&{106}&{117}&{66}&{134}&{138} \\ {118}&{70}&{66}&{53}&{80}&{102} \\ {170}&{122}&{134}&{80}&{157}&{170} \\ {214}&{132}&{138}&{102}&{170}&{213} \end{array}} \right)} \end{array}$ (M1)A1

number of walks of length 4 is 170

Note: The complete matrix need not be shown. Only one of the FE has to be shown.

[2 marks]

Examiners report

[N/A]

d.i.

[N/A]

d.ii.

[N/A]

The following table shows the costs in US dollars (US$) of direct flights between six cities. Blank cells indicate no direct flights. The rows represent the departure cities. The columns represent the destination cities.

The following table shows the least cost to travel between the cities.

A travelling salesman has to visit each of the cities, starting and finishing at city A.

Show the direct flights between the cities as a graph.

[2]

Write down the adjacency matrix for this graph.

[2]

Using your answer to part (b), find the number of different ways to travel from and return to city A in exactly 6 flights.

[2]

State whether or not it is possible to travel from and return to city A in exactly 6 flights, having visited each of the other 5 cities exactly once. Justify your answer.

[2]

Find the values of $a$ and $b$ .

[2]

Use the nearest neighbour algorithm to find an upper bound for the cost of the trip.

[3]

By deleting vertex A, use the deleted vertex algorithm to find a lower bound for the cost of the trip.

[4]

Markscheme

[2 marks]

attempt to form an adjacency matrix M1

$\left( {\begin{array}{*{20}{c}} 0&1&1&0&0&0 \\ 1&0&1&1&1&0 \\ 1&1&0&0&0&0 \\ 0&1&0&0&1&1 \\ 0&1&0&1&0&1 \\ 0&0&0&1&1&0 \end{array}} \right)$ A1

[2 marks]

raising the matrix to the power six (M1)

50 A1

[2 marks]

not possible A1

because you must pass through B twice R1

Note: Do not award A1R0.

[2 marks]

$a = 230$ , $b = 340$ A1A1

[2 marks]

A → B → D → E → F → C → A (M1)

90 + 70 + 100 + 210 + 330 + 150 (A1)

(US$) 950 A1

[3 marks]

finding weight of minimum spanning tree M1

70 + 80 + 100 + 180 = (US$) 430 A1

adding in two edges of minimum weight M1

430 + 90 + 150 = (US$) 670 A1

[4 marks]

Examiners report

[N/A]

The adjacency matrix of the graph G, with vertices P, Q, R, S, T is given by:

$\begin{array}{*{20}{c}} {} \\ {\text{P}} \\ {\text{Q}} \\ {\text{R}} \\ {\text{S}} \\ {\text{T}} \end{array}\begin{array}{*{20}{c}} {\begin{array}{*{20}{c}} {\text{P}}&{\text{Q}}&{\text{R}}&{\text{S}}&{\text{T}} \end{array}} \\ {\left( {\begin{array}{*{20}{c}} 0&2&1&1&0 \\ 2&1&1&1&0 \\ 1&1&1&0&2 \\ 1&1&0&0&0 \\ 0&0&2&0&0 \end{array}} \right)} \end{array}$

Draw the graph of G.

[3]

Define an Eulerian circuit.

[1]

b.i.

Write down an Eulerian circuit in G starting at P.

[2]

b.ii.

Define a Hamiltonian cycle.

[2]

c.i.

Explain why it is not possible to have a Hamiltonian cycle in G.

[3]

c.ii.

Find the number of walks of length 5 from P to Q.

[4]

d.i.

Which pairs of distinct vertices have more than 15 walks of length 3 between them?

[4]

d.ii.

Markscheme

Note: Award A2 for one missing or misplaced edge,

A1 for two missing or misplaced edges.

[3 marks]

an Eulerian circuit is one that contains every edge of the graph exactly once A1

[1 mark]

b.i.

a possible Eulerian circuit is

P → Q → S → P → Q → Q → R → T → R → R → P A2

[2 marks]

b.ii.

a Hamiltonian cycle passes through each vertex of the graph A1

exactly once A1

[2 marks]

c.i.

to pass through T, you must have come from R and must return to R. R3

hence there is no Hamiltonian cycle

[3 marks]

c.ii.

using the adjacency matrix A = ${\left( {\begin{array}{*{20}{c}} 0&2&1&1&0 \\ 2&1&1&1&0 \\ 1&1&1&0&2 \\ 1&1&0&0&0 \\ 0&0&2&0&0 \end{array}} \right)}$ , (M1)

we need the entry in the first row second column of the matrix A⁵ (M1)

A⁵ = $\left( {\begin{array}{*{20}{c}} {245}&{309}&{274}&{143}&{126} \\ {309}&{363}&{322}&{168}&{156} \\ {274}&{322}&{295}&{141}&{164} \\ {143}&{168}&{141}&{77}&{72} \\ {126}&{156}&{164}&{72}&{72} \end{array}} \right)$ (A1)

hence there are 309 ways A1

[4 marks]

d.i.

A³ = $\left( {\begin{array}{*{20}{c}} {13}&{21}&{17}&{10}&6 \\ {21}&{22}&{19}&{11}&8 \\ {17}&{19}&{18}&7&{14} \\ {10}&{11}&7&5&4 \\ 6&8&{14}&4&4 \end{array}} \right)$ (M1)

hence the pairs of vertices are PQ, PR and QR A1A1A1

[4 marks]

d.ii.

Examiners report

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

c.i.

[N/A]

c.ii.

[N/A]

d.i.

[N/A]

d.ii.

Consider the following diagram.

The sides of the equilateral triangle ABC have lengths 1 m. The midpoint of [AB] is denoted by P. The circular arc AB has centre, M, the midpoint of [CP].

Find AM.

[3]

a.i.

Find the area of the shaded region.

[3]

Markscheme

METHOD 1

PC $= \frac{{\sqrt 3 }}{2}$ or 0.8660 (M1)

PM $= \frac{1}{2}$ PC $= \frac{{\sqrt 3 }}{4}$ or 0.4330 (A1)

AM $= \sqrt {\frac{1}{4} + \frac{3}{{16}}}$

$= \frac{{\sqrt 7 }}{4}$ or 0.661 (m) A1

METHOD 2

using the cosine rule

AM² $= {1^2} + {\left( {\frac{{\sqrt 3 }}{4}} \right)^2} - 2 \times \frac{{\sqrt 3 }}{4} \times {\text{cos}}\left( {30^\circ } \right)$ M1A1

AM $= \frac{{\sqrt 7 }}{4}$ or 0.661 (m) A1

[3 marks]

a.i.

EITHER

$\frac{1}{2}{\text{A}}{{\text{M}}^2}\left( {2\,{\text{A}}\mathop {\text{M}}\limits^ \wedge {\text{P}} - {\text{sin}}\left( {2\,{\text{A}}\mathop {\text{M}}\limits^ \wedge {\text{P}}} \right)} \right)$ (M1)A1

$\frac{1}{2}{\text{A}}{{\text{M}}^2} \times 2\,{\text{A}}\mathop {\text{M}}\limits^ \wedge {\text{P}} - = \frac{{\sqrt 3 }}{8}$ (M1)A1

= 0.158(m²) A1

Note: Award M1 for attempting to calculate area of a sector minus area of a triangle.

[3 marks]

Examiners report

[N/A]

a.i.

[N/A]

The diagram shows two circles with centres at the points A and B and radii $2r$ and $r$ , respectively. The point B lies on the circle with centre A. The circles intersect at the points C and D.

N16/5/MATHL/HP2/ENG/TZ0/09

Let $\alpha$ be the measure of the angle CAD and $\theta$ be the measure of the angle CBD in radians.

Find an expression for the shaded area in terms of $\alpha$ , $\theta$ and $r$ .

[3]

Show that $\alpha = 4\arcsin \frac{1}{4}$ .

[2]

Hence find the value of $r$ given that the shaded area is equal to 4.

[3]

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

$A = 2(\alpha - \sin \alpha ){r^2} + \frac{1}{2}(\theta - \sin \theta ){r^2}$ M1A1A1

Note: Award M1A1A1 for alternative correct expressions eg. $A = 4\left( {\frac{\alpha }{2} - \sin \frac{\alpha }{2}} \right){r^2} + \frac{1}{2}\theta {r^2}$ .

[3 marks]

METHOD 1

consider for example triangle ADM where M is the midpoint of BD M1

$\sin \frac{\alpha }{4} = \frac{1}{4}$ A1

$\frac{\alpha }{4} = \arcsin \frac{1}{4}$

$\alpha = 4\arcsin \frac{1}{4}$ AG

METHOD 2

attempting to use the cosine rule (to obtain $1 - \cos \frac{\alpha }{2} = \frac{1}{8}$ ) M1

$\sin \frac{\alpha }{4} = \frac{1}{4}$ (obtained from $\sin \frac{\alpha }{4} = \sqrt {\frac{{1 - \cos \frac{\alpha }{2}}}{2}}$ ) A1

$\frac{\alpha }{4} = \arcsin \frac{1}{4}$

$\alpha = 4\arcsin \frac{1}{4}$ AG

METHOD 3

$\sin \left( {\frac{\pi }{2} - \frac{\alpha }{4}} \right) = 2\sin \frac{\alpha }{2}$ where $\frac{\theta }{2} = \frac{\pi }{2} - \frac{\alpha }{4}$

$\cos \frac{\alpha }{4} = 4\sin \frac{\alpha }{4}\cos \frac{\alpha }{4}$ M1

Note: Award M1 either for use of the double angle formula or the conversion from sine to cosine.

$\frac{1}{4} = \sin \frac{\alpha }{4}$ A1

$\frac{\alpha }{4} = \arcsin \frac{1}{4}$

$\alpha = 4\arcsin \frac{1}{4}$ AG

[2 marks]

(from triangle ADM), $\theta = \pi - \frac{\alpha }{2}{\text{ }}\left( { = \pi - 2\arcsin \frac{1}{4} = 2\arcsin \frac{1}{4} = 2.6362 \ldots } \right)$ A1

attempting to solve $2(\alpha - \sin \alpha ){r^2} + \frac{1}{2}(\theta - \sin \theta ){r^2} = 4$

with $\alpha = 4\arcsin \frac{1}{4}$ and $\theta = \pi - \frac{\alpha }{2}{\text{ }}\left( { = 2\arccos \frac{1}{4}} \right)$ for $r$ (M1)

$r = 1.69$ A1

[3 marks]

Examiners report

[N/A]

In triangle ABC, AB = 5, BC = 14 and AC = 11.

Find all the interior angles of the triangle. Give your answers in degrees to one decimal place.

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

attempt to apply cosine rule M1

${\text{cos}}\,{\text{A}} = \frac{{{5^2} + {{11}^2} - {{14}^2}}}{{2 \times 5 \times 11}} = - 0.4545 \ldots$

$\Rightarrow {\text{A}} = 117.03569 \ldots ^\circ$

$\Rightarrow {\text{A}} = 117.0^\circ$ A1

attempt to apply sine rule or cosine rule: M1

$\frac{{{\text{sin}}\,117.03569 \ldots ^\circ }}{{14}} = \frac{{{\text{sin}}\,{\text{B}}}}{{11}}$

$\Rightarrow {\text{B}} = 44.4153 \ldots ^\circ$

$\Rightarrow {\text{B}} = 44.4^\circ$ A1

${\text{C}} = 180^\circ - {\text{A}} - {\text{B}}$

${\text{C}} = 18.5^\circ$ A1

Note: Candidates may attempt to find angles in any order of their choosing.

[5 marks]

Examiners report

[N/A]

In a triangle ${\text{ABC, AB}} = 4{\text{ cm, BC}} = 3{\text{ cm}}$ and ${\rm{B\hat AC}} = \frac{\pi }{9}$ .

Use the cosine rule to find the two possible values for AC.

[5]

Find the difference between the areas of the two possible triangles ABC.

[3]

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

METHOD 1

let ${\text{AC}} = x$

${3^2} = {x^2} + {4^2} - 8x\cos \frac{\pi }{9}$ M1A1

attempting to solve for $x$ (M1)

$x = 1.09,{\text{ }}6.43$ A1A1

METHOD 2

let ${\text{AC}} = x$

using the sine rule to find a value of $C$ M1

${4^2} = {x^2} + {3^2} - 6x\cos (152.869 \ldots ^\circ ) \Rightarrow x = 1.09$ (M1)A1

${4^2} = {x^2} + {3^2} - 6x\cos (27.131 \ldots ^\circ ) \Rightarrow x = 6.43$ (M1)A1

METHOD 3

let ${\text{AC}} = x$

using the sine rule to find a value of $B$ and a value of $C$ M1

obtaining $B = 132.869 \ldots ^\circ ,{\text{ }}7.131 \ldots ^\circ$ and $C = 27.131 \ldots ^\circ ,{\text{ }}152.869 \ldots ^\circ$ A1

$(B = 2.319 \ldots ,{\text{ }}0.124 \ldots$ and $C = 0.473 \ldots ,{\text{ }}2.668 \ldots )$

attempting to find a value of $x$ using the cosine rule (M1)

$x = 1.09,{\text{ }}6.43$ A1A1

Note: Award M1A0(M1)A1A0 for one correct value of $x$

[5 marks]

$\frac{1}{2} \times 4 \times 6.428 \ldots \times \sin \frac{\pi }{9}$ and $\frac{1}{2} \times 4 \times 1.088 \ldots \times \sin \frac{\pi }{9}$ (A1)

( $4.39747 \ldots$ and $0.744833 \ldots$ )

let $D$ be the difference between the two areas

$D = \frac{1}{2} \times 4 \times 6.428 \ldots \times \sin \frac{\pi }{9} - \frac{1}{2} \times 4 \times 1.088 \ldots \times \sin \frac{\pi }{9}$ (M1)

$(D = 4.39747 \ldots - 0.744833 \ldots )$

$= 3.65{\text{ (c}}{{\text{m}}^2})$ A1

[3 marks]

Examiners report

[N/A]

Boat A is situated 10km away from boat B, and each boat has a marine radio transmitter on board. The range of the transmitter on boat A is 7km, and the range of the transmitter on boat B is 5km. The region in which both transmitters can be detected is represented by the shaded region in the following diagram. Find the area of this region.

Markscheme

* This question is from an exam for a previous syllabus, and may contain minor differences in marking or structure.

use of cosine rule (M1)

CÂB = arccos $\left( {\frac{{49 + 100 - 25}}{{2 \times 7 \times 10}}} \right) = 0.48276 \ldots \left( { = 27.660 \ldots ^\circ } \right)$ (A1)

C $\mathop {\text{B}}\limits^ \wedge$ A = arccos $\left( {\frac{{25 + 100 - 49}}{{2 \times 5 \times 10}}} \right) = 0.70748 \ldots \left( { = 40.535 \ldots ^\circ } \right)$ (A1)

attempt to subtract triangle area from sector area (M1)

area $= \frac{1}{2} \times 49\left( {2{\text{C}}\mathop {\text{A}}\limits^ \wedge {\text{B}} - {\text{sin}}\,{\text{2C}}\mathop {\text{A}}\limits^ \wedge {\text{B}}} \right)\, + \frac{1}{2} \times 25\left( {2{\text{C}}\mathop {\text{B}}\limits^ \wedge {\text{A}} - {\text{sin}}\,{\text{2C}}\mathop {\text{B}}\limits^ \wedge {\text{A}}} \right)$

= 3.5079… + 5.3385… (A1)

Note: Award this A1 for either of these two values.

= 8.85 (km²) A1

Note: Accept all answers that round to 8.8 or 8.9.

[6 marks]

Examiners report

[N/A]